
For H– atom first excitation state is = n 2 i.e., for H-atom n 2, n 3, n 4 are excited state. (ii) In excited state : Energy levels greater than n 1 are excited state. In ground state energy of atom is minimum and for 1 st orbit of H-atom, n=1. (i) In ground state : No energy emission.
This can be represented as where, is known as Rydberg constant. When an electron jumps from an outer orbit (higher energy)n 2 to an inner orbit (lower energy)n 1 then the energy emitted in form of radiation is given by The energy decreases as the value of atomic number Z increases. V ∝ Z Thus, we have H He + > Li +2 > Be +3 (b) For a particular orbit of different species (iii) Calculation of velocity of electron Where, n = Orbit number, m =Mass number 9.1 × 10 −31/kg = Charge on the electron 1.6 × 10 −19 Z = Atomic number of element, k = Coulombic constant 9 × 10 9 Mn 2c −2Īfter putting the values of m, e, k, h, we get. (ii) Calculation of radius of Bohr’s orbit : According to Bohr, radius of orbit in which electron moves is (i) Bohr’s theory satisfactorily explains the spectra of species having one electron, viz. It has to fall back to a lower orbit with the release of energy. When an electron absorbs energy, it gets excited and jumps to an outer orbit. (viii) The lowest energy state ( n = 1) is called the ground state. Hence the spectrum of the atom will have certain fixed frequency.

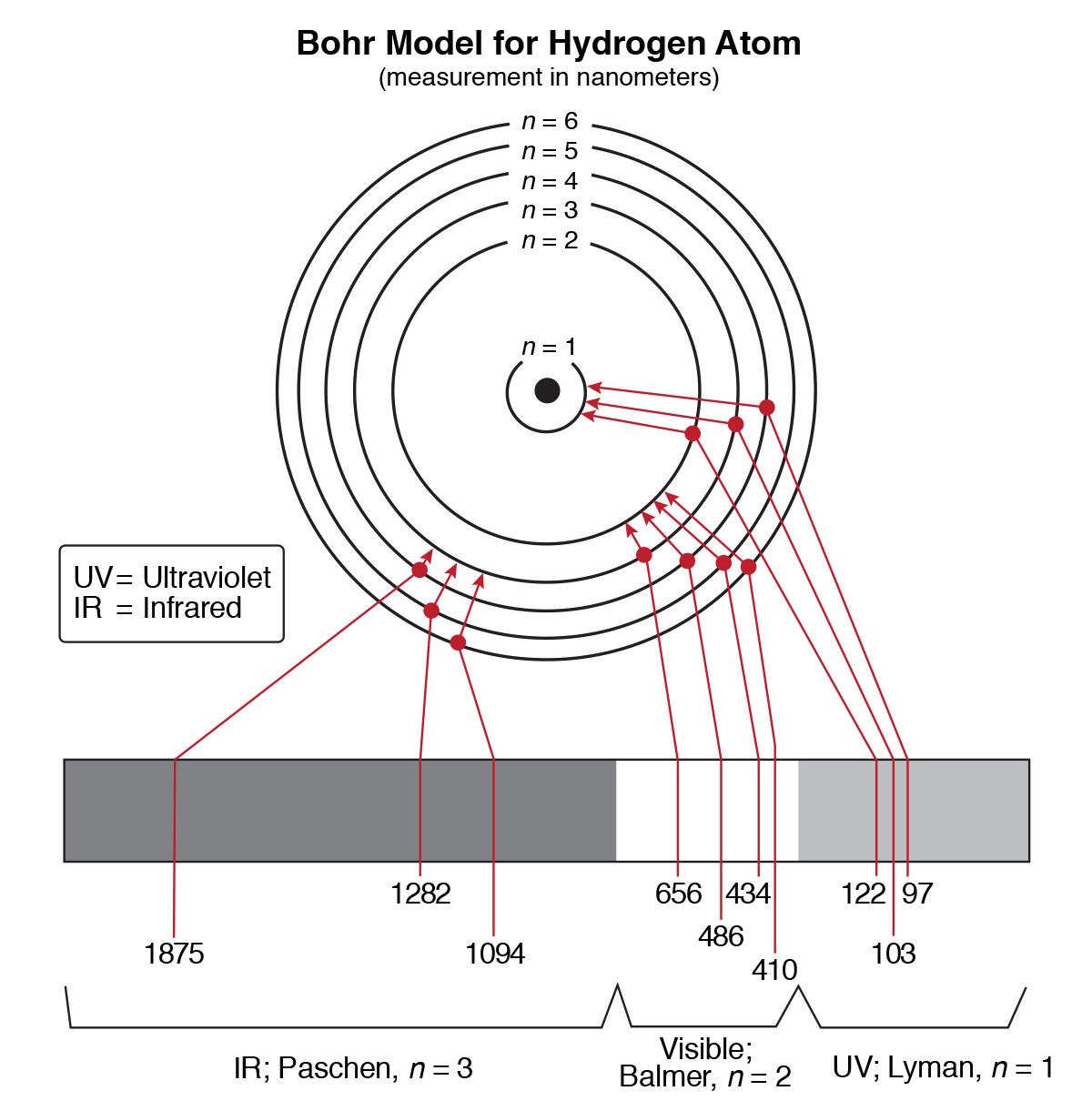
Where ‘ h’ =Planck’s constant, v = frequency of the radiant energy. (vii) The radiation is emitted or absorbed as a single quantum (photon) whose energy hv is equal to the difference in energy ΔE of the electron in the two orbits involved. (vi) The emission or absorption of radiation by the atom takes place when an electron jumps from one stationary orbit to another. Since the electron present in these orbits is associated with some energy, these orbits are called energy levels. Various energy levels are designed as K ( n = 1), L( n = 2), M( n = 3) - etc.
It can have the values n = 1, 2, 3, - (from the nucleus). In the above equation ‘n’ is any integer which has been called as principal quantum number. (v) The angular momentum can be This principal is known as quantization of angular momentum. Where, m = mass of the electron, r = radius of the electronic orbit, v = velocity of the electron in its orbit. (iv) An electron can move only in those permissive orbits in which the angular momentum (mvr) of the electron is an integral multiple of h/2π Thus, (iii) The force of attraction between the nucleus and the electron is equal to centrifugal force of the moving electron.įorce of attraction towards nucleus = centrifugal force Such orbits are known as stable orbits or non – radiating or stationary orbits. (ii) The electron in an atom revolve around the nucleus in certain discrete orbits. (i) The atom has a central massive core nucleus where all the protons and neutrons are present. It gave new idea of atomic structure in order to explain the stability of the atom and emission of sharp spectral lines. (1) This model was based on the quantum theory of radiation and the classical law of physics.


 0 kommentar(er)
0 kommentar(er)
